Statins and death postponement

Applied statistics belongs to the same field than Magic. Hence it is marvelous whether you are aware of the trick or not.

A. P.

Before anything else. Everyone of us is going to die. I don't think there is any need to play with the information available about statins to give them a bad appearance. They do already have it whenever we do a minimally critical analysis. Hence this post.

I will introduce computing methods that can require some time to understand. Feel free to skip over them.

Prologue

First an example by Vicente.

Suppose your boss is giving an extra payment to all of her employees. Suppose it is being given at different times along the current year. Suppose she gives a different quantity to each one of her employees. Suppose a month has already passed and you have access to the following questions and their answers:

1. How much is the average extra payment already received by all the employees? Adding all the extra payments already given and dividing by the number of all the employees gives us the average amount received by anyone and it equals 20€.
2. How much is the average extra payment already received by those who have received it? Let's say 20% of your colleagues has already received the extra payment and the average for them has been of 100€. It is compatible with Point 1 because there are an 80% of employees that have received 0€ at the time being:

   0.2 • 100€ + 0.8 • 0€ = 20€.

3. How much is the average extra payment going to be? You know the assistant of your boss and he has heard her saying that the average extra payment is going to be of 80€.

The paper I comment in this post answers a question similar to that of Point 1. I think we all consider more useful an answer to Point 3. If there is not enough information available we could settle for an answer to Point 2 though.

Statins and death postponement

A Danish research group has recently published a paper where they compute how much longer within the trial duration time all of those who take statins live with respect to a control group who don't (they either take placebo, nothing at all or usual care treatment). They compute this gain for every statin with control group trial publishing enough information, that is, including all-cause mortality curves.

Actually it was Dr. Kendrick who first pointed out that lives are not being saved but merely death is slightly posponed. He was unable to publish it in the peer reviewed literature though. Go figure.

Dr. Kendrick has written a post about the paper by Kristensen et alter^* too and I have commented there a highly condensed version of what I am going to explain here.

I write this post because I have read conclusions about the paper that doesn't follow at all from it (confusing aswer to Question 1 with the one to Question 3). I am going to replicate the method by Kristensen et alter^* first. Afterwards I am going to extend it to answer what people is really interested in (Question 3). Finally I am going to explain why this last step doesn't make sense.

Method by Kristensen et alter^*

The question they answer is: what is the average death postponement time all the statin consumers acquire with respect to those not taking them during the trial duration time? Its details are important so I excerpt their paper at its Discussion Section, second paragraph (my bolds):

First, this analysis only estimates the survival gain achieved within the trials’ running time. After termination of the trials, the treated would continue to accrue survival gain as long as there was a difference in cumulative mortality between the treatment arms.

They don't include the word "average" because another different estimator is used.


How do they compute this survival gain? They explain their method in the Web Appendix. I am going to explain it here replicating it for the same LIPID trial. I will include some explanatory graphs.


Kristensen et alter^* start with the published survival curves for both those under statins and those under placebo:

This plot has a mortality range from 0% to 15%. It has a time range from 0 to 7 years. At 6.1 years mortality under statins arrives to 11% and under placebo to 14.1%.


I perform the following steps from this initial figure:

1. The area M_K between the two mortality curves is computed. I color it magenta. This time we have M_K = 17.4 cm².
2. An auxiliar area A is computed.  It represents some arbitrary time (t=2 years in this case) for some arbitrary fraction of subjects (10% in this case). I color it yellow. This time we have A = 56'84 cm².
3. This auxiliar area is implicitly expanded to a reference area T_K by Kristensen et alter^* in order to represent the 100% of subjects by dividing by its height (10%). I color it cyan. This time we have T_K = A/(10%) = 568.4 cm².
   
4. Finally a rule of three is performed. We know the reference area (T_K) represents two years (t) for all the subjects. We compute how much average extra life time (m_K) during the 6.1 years of duration of the trial is represented by the area between curves (M_K) for everyone under statins. This time we have m_K = M_K • t / T_K = 17.4 • 2 /  568.4 = 0.06122 years  • 365 days / (1 year) = 22.35 days.

In this same case Kristensen et alter^* compute an average death postponement for everyone under statins during the duration of the trial of 22.07 days. Close enough. I consider validated my implementation of their method.

Explanation

It is easier to understand what the method is doing if we think in exactly N=100 subjects in each one of the two arms. Every time a subject under placebo dies we measure the time until either the corresponding subject under statins dies too or we reach the trial end at 6.1 years. This way we add for each subject under placebo (dead or alive at trial end) the amount of extra time the corresponding subject under statins has lived till either dead or arriving alive at the trial end.

As time goes by we have:

• The first two either under statins or placebo die more or less at the same time. Those are the two magenta points surrounding year 1.
• From the third to the eleventh subject under placebo die before the one under statins. This gives rise to segments of 0.4, 0.9, 1.5, 1.7, 1.6, 1.7, 1.9, 2.2 and 2.3 cm.
• From the twelfth to the fourteenth subject under placebo die while those under statins are still alive at 6.1 years. This gives rise to segments of 1.7, 1.1 and 0.3 cm.
• From the fifteenth to the one hundredth there will be no difference in survival because all of them arrive alive to trial end at 6.1 years. I don't plot these points.

How do we estimate the average death postponement for everyone (death or alive) under statins during the 6.1 years of the trial?:

• We measure how long in centimeters is t = 2 years in the figure to compute the cyan reference piece. This time it measures 6 cm, that is, 3 cm each year. We approximate the cyan reference piece adding 100 times (shown the first 17 segments and ellipsis afterwards) this 6 cm segment: T_K = N • 6 = 100•6 = 600 cm.
• The previous area between curves here is approximated by the sum of extra live of those under statins with respect to those under placebo (I omit the 87 zeros): M_K = 0.4 + 0.9 + 1.5 + 1.7 + 1.6 + 1.7 + 1.9 + 2.2 + 2.3 + 1.7 + 1.1 + 0.3 = 17.3 cm (that is, 17.3/3 = 5.77 extra years accrued by all subjects under statins in this 6.1 years).
• Finally we apply the rule of three in order to compute how much extra live time share belongs to each subject under statins: m_K = M_K • t / T_K = 17.3 • 2 / (N • 6) = (17.3/3)/N = 5.77 years / 100 = 0.0577 years • 365 days / (1 year) = 21.05 days.

Dividing by the cyan area is equivalent to change from length to time and concurrently computing an average dividing by the number of subjects under statins.

We should assign a height in centimeters for each subject (line separation is 1 cm in this case) and multiply by it in order to have actual areas (cm² instead of cm) for approximations M_K and T_K. It won't impact the result since M_K is divided by T_K though.

If we would increase the number of subjects from N=100 then the result would approach from 21.05 days to the 22.34 days computed directly with areas.

Finally, it may happen that the trial doesn't have an actual N value. It is clearly seen when trials are stopped early. Since subjects are not recruited at the same time, there will be a lower number of subjects as we look at a more advanced time.

Analysis limitations

The main drawback of the results by Kristensen et alter^* is the lack of confidence intervals. Without them we can't be sure how much close we are to the real value we are estimating. I don't know if there is enough information in the published trial studies to compute them though.

There are more problems when trying to draw conclusions from these results:

• Those still alive under placebo when the trial ends make their corresponding subjects under statins contribute with ZERO to the extra life computation (just like those that haven't received the extra payment yet at the prologue).
• Kristensen et alter^* doesn't answer the following question: how much extra time is going to live someone when taking statins for 6.1 year (or until death if it happens first)? This will need extrapolations to be made but it is the actual question people think is being addressed.
• There are doubts about convergence of mortality curves like in the JUPITER trial. There are those that think that sooner or later both curves will cross and subjects under statins will begin to die ealier than subjects under placebo.

  

  Source: Spanish critique to the JUPITER trial by López & Wright

  Kristensen et alter^* already talk about this issue (same paragraph than before):

  There are a few studies with long-term follow-up after cardiovascular intervention trials showing that this survival might be substantial,¹⁷ but there are also studies showing that mortality becomes similar in the two groups after the trial’s termination.¹⁸

  These studies follow both arms when the trial ends and everyone is under statins. It seems that this kind of studies are not very popular though.
• Not being possible to answer the question people are interested in, I think we should answer the one we can: how much extra time have lived those dead under statins during the trial because of the treatment?

I will try to answer this latter question. Afterwards I will try to do a conservative estimate of the former.

Extra time lived by those dead under statins during the trial

This time we focus on a shorter area where we can compute all of the extra time lived by those dead under statins during the trial. The magenta area goes only till the horizontal line with 11% of deaths (mortality under statins during the trial). We have M = 14.05 cm².

We have a cyan area representing 2 years of life for exactly this 11% of subjects and not the 100%. We have T = 63'8 cm².

The average time of extra life of those dead under statins (11%) with respect to those under placebo is m = M • t / T = 14.05 • 2 / 63.8 = 0.4404 years • 365 days / (1 year) = 160.8 days (about 5 months).

Explanation

Let's come back to the "N=100 subjects" approximation. Now we are computing only the extra life time achieved by those dead under statins with respect to those under placebo.

As time goes by we will have only the first two groups in this case:

• The first two either under statins or placebo die more or less at the same time. Those are the two magenta points surrounding year 1.
• From the third to the eleventh subject under placebo die before the one under statins. This gives rise to segments of 0.4, 0.9, 1.5, 1.7, 1.6, 1.7, 1.9, 2.2 and 2.3 cm.

This time we share the extra life achieved only among the n=11 dead under statins and not among the total number N=100 of subjects in each arm:

• We have that t = 2 years still measures 6 cm, that is, 3 cm each year. We approximate the cyan reference piece adding n=11 times this 6 cm segment: T = n • 6 = 11•6 = 66 cm.
• The previous area between curves here is approximated by the sum of extra life of those under statins with respect to those under placebo (zeroes included this time): M = 0 + 0 + 0.4 + 0.9 + 1.5 + 1.7 + 1.6 + 1.7 + 1.9 + 2.2 + 2.3 = 14.2 cm (that is, 14.2/3 = 4.73 extra years accrued by those dead under statins during this 6.1 years).
• Finally we apply the rule of three in order to compute how much extra life time share belongs to each subject dead under statins: m = M • t / T = 14.2 • 2 / (n • 6) = (14.2/3)/n = 4.73 years / 11 = 0.4303 years • 365 days / (1 year) = 157.06 days.

Dividing by the cyan area is equivalent to change from length to time and concurrently computing an average dividing by the number of subjects dead under statins during the trial.


If we would increase the number of subjects from N=100 then n would increase too and the result would approach from 157.06 days to the 160.8 days computed directly with areas.

Statistics of interest

We may decompose the benefit attained during the trial duration due to taking statins for 6.1 years instead of placebo into:

• 11% have died after living an average of 160.8 extra days.
• 14.1%-11%=3.1% are still alive while they would be dead under placebo. This is the absolute risk reduction. There are other two related statistics:
  • Inverting it we get the NNT (Number Needed to Treat): number of subjects under treatment so one of them gets the benefit (still alive at 6.1 years due to treatment), 100/3.1=32.26.
  • The ratio between mortality under treatment and under placebo gives rise to relative risk of dying: 11/14.1 = 78%. If we substract this quantity from the unity we get the relative risk reduction: 1-11/14.1 = 3.1/14.1 = 22%. These pair of statistics are useful to answer the pair of questions about the hypothetical situation where I die within the first 6.1 years due to not taking statins:
    • What would be the probability of still being alive (some months added so I would make it to 6.1 years) if I would have taken them? The answer is the relative risk reduction, that is in this case, 22%.
    • Even more interesting, what would be the probability of me dying within those first 6.1 years anyway? The answer is the relative risk, that is, 1-22%=3'1/14'1=78%.
    I hope you see the tiny efficacy of the treatment with statins even in favorable trials like LIPID.
• We have 100%-14.1%=85.9% that hasn't get any benefit in added life within these 6.1 years because they would be alive anyways.

We may relate all of these statistics to the NNT. For every 32.26 subjects taking statins for 6.1 years we get:

• 1 saved,
• 32.26•11% = 3.55 dead anyways after living an average 160.8 extra days.
• 32.26•(1-14.1%) = 27.71 survivors independent of treatment.

Collapsing these statistics to the average life extension for everyone on statins (dead or alive at the trial end) is losing a lot of useful information.

Extra time lived by those dead under statins during the trial: Approximation

You can call me lazy. I haven't replicated all of the analysis for each and every one of the trials studied by Kristensen et alter^*. Instead I have estimated approximately how much extra time have lived those dead under statins during the trial duration time working from their estimation up.

Let's suppose that the area between curves would include not only the magenta one computed by Kristensen et alter^* but the hypothetical green one (G) of extra live for those extra still alive under statins with respect to placebo at trial end (14.1%-11%=3.1%). Then we could compute the extra time lived by those dead under treatment simply changing the reference area from the 100% height used by Kristensen et alter^* (T_K) to that cyan area taking into account only the 14.1% of subjects: T_ext = T_K • 14.1% . Hence we could get the result we are interested in just by dividing by 14.1%:

m_ext = (M_K+G) • t / T = (M_K+G) • t / (T_K•14.1%).

Since we actually don't know how much the green area would measure, our approximation (m_app) will be actually lower than this value:

m_app = M_K • t / (T_K•14.1%) =  m_K/(14.1%)< m_ext.

It is expected to be lower than focusing on only the first 11% of those dead under statins too.

If we check this approximation for the LIPID trial we get the approximation:

m_app = 22.05/0.141 = 156.4 days

We had m=160.8 extra days for those first 11% dead under statins in the previous section. We see that the approximate value is close enough.

If we compute this approximate value for those trials studied by Kristensen et alter^* we have:

• Secondary prevention:
  • 4S (4444 subjects, year 1994, statin vs placebo): 27.18/0.123 = 221.0 extra days for the first 8.7% dead under statins during the trial 5.8 years.
  • LIPID (9014 subjects, year 1998, statin vs placebo): 22.05/0.141 = 156.4 ( 160.8) extra days for the first 11% dead under statins during the trial 6.1 years.
  • GISSI-P (4271 subjects, year 2000, statin vs no treatment): 1.76/0.0413 = 42.6 extra days for the first 3.37% dead under statins during the trial 2 years.
  • CORONA (5011 subjects, year 2007, statin vs placebo): 4.09/0.304 = 13.5 extra days for the first 29% dead under statins during the trial 2.7 years.
  • GISSI-HF (4631 subjects, year 2008, statin vs placebo): -9.51/0.288 = -33.0 → 33.0 extra days for the first 28.1% dead under placebo during the trial 4.4 years.
• Primary prevention:
  • High risk of bias (clearly explained by the Therapeutics Initiative):
    • ASCOT-LLA (19342 subjects, year 2003, statin vs placebo): 1.99/0.041 = 48.5 extra days for the first 3.6% dead under statins during the trial 3.5 years.
    • CARDS (2838 subjects, year 2004, statin vs placebo): 18.66/0.058 = 321.7 extra days for the first 4.3% dead under statins during the trial 4.8 years.
    • MEGA (7832 subjects, year 2006, statin vs no treatment): 4.42/0.0166 = 266.3 extra days for the first 1.11% dead under statins during the trial 5 years.
    • JUPITER (17802 subjects, year 2008, statin vs placebo): 7.26/0.0277 = 262.1 extra days for the first 2.22% dead under statins during the trial 4 years —the median duration was 1.9 years.
  • Low risk of bias:
    • WOSCOPS (6595 subjects, 44% smokers, year 1995, statin vs placebo): 9.33/0.041 = 227.6 extra days for the first 3.2% dead under statins during the trial 5 years.
    • ALLHAT-LLT (10355 hypertensive subjects, year 2002, statin vs usual care): -4.96/0.153 = -32.4 → 32.4 extra days for the first 15.3% dead under usual care (14.9% dead under statins) during the trial 6 years.

Yes, negative number means there was a tendency to die first under statins. In this case we divide by the maximum mortality of both arms. That happened under statins in GISSI-HF. It happened under usual care in ALLHAT-LLT:

Source: ALLHAT-LLT

ALLHAT-LLT was the only big trial independently funded. That's no small fact but if you think it doesn't matter who funds the research you should start reading about trial 329. Aferwords you may read about Vioxx (another article about it). Finally much more information available in some books such as Deadly Medicines and Organised Chrime, Doctoring Data or Bad Pharma.

Due to Vioxx and after 2004 there was some regulations changes in USA and EU too. Internationally there has been similar pressures such as mandatory registration of trials before recruiting subjects in order to be publishable afterwards. Do these facts have any impact in trial results? Judge by yourself:

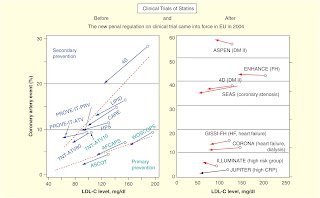Source: Statins stimulate atherosclerosis and heart failure: pharmacological mechanisms (via Vicente)

There are other clues pointing out to potential data massage as stated by Sir Richard Thompson et alter^*:

Furthermore, the rate of adverse effects in the statin and placebo arms of all the trials has been almost identical. Exact comparison between trials is not possible, due to lack of complete data, and various measures of adverse effects are used, in different ways.

A common source of bias taken into account by the Therapeutics Initiative in its meta-analysis is stopping trials early because it introduces a robust relative risk of about 0.71 with respect to those driven toward completion. This practice has been highly critized some time ago so ignorance about it should be already scarce. Doing as if nothing happens and using data from such a trial in a meta-analysis completely invalidates its conclusions. I can only think of two possible reasons to do so: either malice or incompetence in statistics.

Given all of these details, what trials should be more reliable? I have already highlighted them in bolds:

• Secondary prevention: tentatively GISSI-HF  and CORONA, just because they have been published after 2004. I haven't checked either if they were stopped early or if they published intention to treat analysis (source of bias in the MEGA trial) though.
• Primary prevention: ALLHAT-LLT, because it was funded independently from Big Pharma.

Extrapolated life-long life extension due to statins

We are going to do an extrapolation toward the future in order to estimate how much those taking statins would live with respect to not taking them. We are going to suppose both no further benefit and no further damage from them. This supposition would be somewhat more plausible when subjects stop treatment after completion of the trial.

The conservative (supposing not further benefit nor harm) approach would be that both 1-0.11=89% survivors on placebo at 5.2 years and 89% survivors on statin at 6.1 year started dying exactly at the same rate. I have measured (red segment over the magenta area) something like 2.4 cm • year/(3 cm) • 365 days/(1 year) = 292 days between those two survival curves when they arrive at 11% (similar method as Dr. Kendrick's). That would be the conservative estimation of death postponement for all of those 89% still alive under treatment at 5.2 years. That way the conservative average gain for anyone under treatment would be:

0.11 x 160.8 + (1-0.11) x 292 = 277.6 days.

Of course it gets really interesting when focusing on the big study not funded by Big Pharma (ALLHAT-LLT) though. I dare you to do a reasonable extrapolation given their two mortality curves already shown.

Analysis limitations

I think the previous method used to extrapolate outcomes is minimally plausible whenever the treatment duration time is shorter enough than the outcome measurement time. This happens in treatments like chemotherapy (tamoxifen included). That's not the case here where treatment is supposed to be even chronic. Even if all statin trials gave consistent results, I don't think it would be a good idea to try to extrapolate.

Source: Stats Land

There are some secondary effects of statins that are going to increase as time goes by such as worse blood glucose control. It is going to be a matter of time that it brings about its consequences.

Source: Statins stimulate atherosclerosis and heart failure: pharmacological mechanisms

I think that in statins case focus should be on all of those statistics shown earlier and not on extrapolations.

Conclusion

Is there intelligent life outside the blood cholesterol hypothesis? You bet.

Take a look at the graph (and full post) of Ivor Cummings:

Source: Atherosclerosis Root Cause Diagram with References

Perhaps reducing blood cholesterol levels and doing nothing else can give you some extra months without a heart attack. There are those who think that considering such a meager, shubby, negligible approach just one to be ignored in order to keep searching is being a cholesterol/statin denier. Sorry, being engineers doesn't make us stupid. I don't think focusing on cholesterol is a too bright approach.

[Added on January 14]
You should take a look at Zahc's take on the cholesterol issue on his slide show.

There are those who have checked the efficacy of a low carb diet plus vitamin D supplemenation in suppressing coronary events in complying (secondary prevention) patients.

My numbers at June 23:

• Total cholesterol: 316 mg/dl.
• HDL cholesterol: 91 mg/dl.
• Tryglycerides: 76 mg/dl.

I am not betting my health on the advice of those focusing on cholesterol and issuing inconsistent guidelines. I am not waiting till more evidence is accrued. I have done a benefit-risk analysis and proceed with a low carb diet and vitamin D (plus K2) supplementation.

You can do as you wish. And if you are going to discontinue statins better abide to Dr. Graveline's advice: do it slowly.

[Added on January 14]
As pointed out by Mie the peer reviewed paper talking about the risk of abrupt statin discontinuation is the one by Drs. Endres & Laufs.

Epilogue

A police officer sees a drunken man intently searching the ground near a lamppost and asks him the goal of his quest. The inebriate replies that he is looking for his car keys, and the officer helps for a few minutes without success then he asks whether the man is certain that he dropped the keys near the lamppost.

“No,” is the reply, “I lost the keys somewhere across the street.” “Why look here?” asks the surprised and irritated officer. “The light is much better here,” the intoxicated man responds with aplomb.

Source: “Did You Lose the Keys Here?” “No, But the Light Is Much Better Here” 

I don't think they are going to find the cure for cardiovascular disease while they stubbornly keep looking for it where the money shines: plain cholesterol reduction.

*[Added on 11/7/2023] I am not fond at all of abbreviations and acronyms. I have been trying to use the complete form of et al. but I was using a complete incorrect one as Athaic has pointed out to me recently. Since it is not clear to me if the neutral one (et alia) is correct in every case or not (there are the masculine et alii and femenine et aliae too) I will surrender and will use the abbreviation until I am certain. One inconvenience still persisting will be that it should not be read aloud without using the complete form or translating it simultaneously to English.